The area of a new class of semi-regular triangle (eutrigons) in etu
This web page is quoted from (or largely based on) the author's book Principles of nature; towards a new visual language, WA Roberts P/L Canberra. 2003. [Used with permission of the author]
Beginning with our defined unit (etu), and having obtained an expression for the area of the most symmetric of triangles (the equilateral triangle) in terms of our defined unit (p2 etu), we are now well-placed to find an expression for the areas of less-regular triangles [yet also] expressed in etu’s.
A new class of triangle defined: the eutrigon
We begin by considering the area of an important semi-regular triangle [in fact an important new class of triangle], the analogue of the right-triangle in orthogonal (Cartesian) coordinate geometry. This triangle has, instead of one angle equal to 90 degrees, one angle equal to 60 degrees. Let us call such a triangle, a eutrigon.
 |
|
| A eutrigon is a triangle with one angle equal to 60 degrees. |
|
|
|
Mini-movie with narration, 72Kb:
The area of a eutrigon in etu
This neglected, hitherto unnamed, and unclassified triangle-type is a very special class of triangle, as we shall see later. But for now we seek to find an expression for the area of any eutrigon given in etu .
Let triangle ABC be any eutrigon. We define C to be the 60o angle, a and b to be the ‘legs’ or sides adjacent to C, and c the ‘hypotenuse’ or side opposite angle C (fig. 56).
Figure 56
Example of a eutrigon
We shall form a geometric construction around the eutrigon in order to determine its area in [terms of] etu’s. The reasons for this will become apparent, but suffice to say that scale-structure theory hints at relating such a form to a 'resonant' symmetric whole.
We proceed as follows:
Let the unknown area (in terms of etu) of eutrigon ABC be Q.
Make a copy of eutrigon ABC and place this hypotenuse-to-hypotenuse with the first to form a parallelogram ADBC. (Fig. 57)
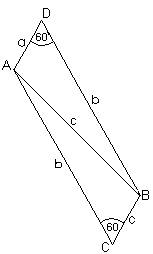
Figure 57
The area of the parallelogram ADBC is simply 2Q since it consists of two identical eutrigons each of defined area Q.
The reasoning behind this approach is that it creates a resonant scalar construction of unequal parts which together form a symmetrical whole, or ‘scale’ (much like completing an octave in music).
Now construct centrifugal (outward pointing) equilateral triangles on the legs of one of the component eutrigons as in figure 58...
Figure 58
It can be seen from an examination of figure 58, that the construction has formed a large equilateral triangle DEF. Also we note from figure 58 that, in terms of areas,
DEF = ACE + BCF + 2Q
Applying our knowledge that the area of an equilateral triangle in etu is simply the side-length 'squared', or p2... we observe that ACE and BCF are each equilateral triangles of side-lengths b and a respectively, and therefore their areas, expressed in terms of etu’s, are given by b2 and a2 respectively.
Thus, [the] equation [above], expressed in etu, becomes:
Area of DEF = a2 + b2 + 2Q
But DEF is equilateral and therefore its area in etu’s is its side-length 'squared'. Its side-length can be seen from an inspection of fig. 58 to be simply (a + b). Thus the area of DEF in etu is (a + b)2 . Substitution in the equation [immediately above] gives
(a + b)2 = a2 + b2 + 2Q
[Scale structure theory implies that there must be resonances or consonances between geometric scales and number scales (including the more generic numbers of algebra). Any student of algebra, learns very early on that the expansion of the expression (a + b)2 is equal to a2 + 2ab + b2. See footnote for a scale-structural visual proof of this, but this time using the traditional square areas with which we are all so familiar]
Thus we accept that the term (a + b)2 is again equal to a2 + 2ab + b2, including triangular systems of analytic geometry. Thus, upon substitution of the expanded version of (a + b)2 in our equation above, we now have,
a2 + 2ab + b2 = a2 + b2 + 2Q
After cancelling corresponding terms on the left and right-hand sides of the above equation, we have simply:
2ab = 2Q
dividing both sides by 2, this equation reduces to the elegant identity [expressed in terms of relative units, in this case, etu],
areaQ = ab
Thus, the area of any eutrigon expressed in etu is ab, where a and b are the lengths of its legs (namely those sides adjacent to the 60-degree angle, as illustrated in fig. 59),
Areaeutrigon = ab
Figure 59
Visual proof that (a + b)2 is equal to a2 + 2ab + b2
email author: contact/feedback
| back to top |
next > |